Contents.
Childhood.
The German mathematician Georg Friedrich Bernhard Riemann was born on September 17, 1826 in Breselenz, Germany. Georg was a son of Friedrich Riemann who was a poor Lutheran minister in Breselenz; Charlotte Ebell was Georg’s mother who died when Georg was 20 years of age [3]. Of Friedrich’s six children, only Georg and one of his many sisters survived into adulthood; however, Georg suffered from perpetually poor health [3]. Georg was predominantly introverted; his composure appeared shy due to of his intense fear of public speaking [1, 4]. Georg’s father instructed Georg in much of his elementary education; at a young age, Riemann demonstrated a great aptitude for mathematics [1].
At 10 years of age, a local school-teacher named Schulz recognized Riemann abilities; Schulz lent Riemann advanced mathematics books to assist in his education [1, 3]. In 1840, Riemann moved to Hanover to reside with his grandmother where he attended the Lyceum middle-school; at the Lyceum, Riemann was admitted directly into the third-class where he studied philosophy and theology in order to become a priest [1, 3]. Outside of his studies, Riemann’s interests in mathematics consumed most of his activities; his ability to solve complex problems astonished many of his instructors. In 1842, the death of Riemann’s grandmother prompted him to transfer to the Johanneum high-school in Luneburg [3].
University Studies.
In the spring of 1846, Riemann enrolled in the University of Gottingen where he was instructed in mathematics by Carl Friedrich Gauss; Gauss encouraged Riemann to pursue a degree in mathematics [4]. As a result, Riemann transferred to the University of Berlin in 1847 to study mathematics under the instruction of Lejeune Dirichlet and Gotthold Eisenstein [3, 4]. In particular, Dirichlet enjoyed making theories intuitive by employing non-rigorous logical analysis; Riemann adopted these methods in his works which resulted in many of his conclusions being rigorously unsatisfactory [3]. During his studies at the university, Riemann formulated his theory of complex variables; moreover, he consulted with Eisenstein about applying complex variables in the theory of elliptic functions [3].
Graduate Studies.
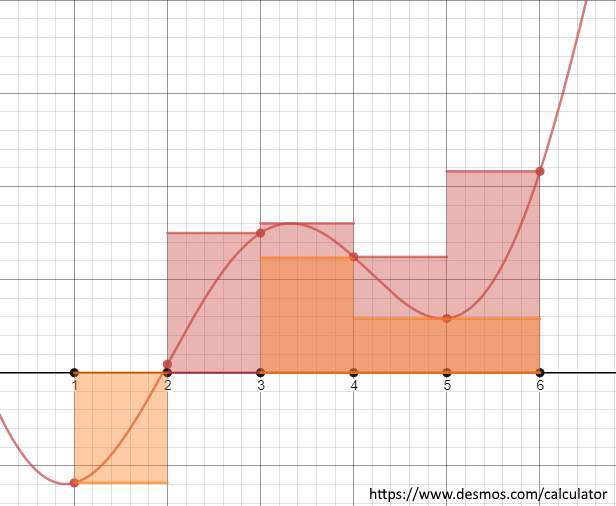Riemann graduated from the University of Berlin in 1849 [1]. Afterward, he returned to the University of Gottingen to engage in doctoral studies under the supervision of Gauss [1]. At the university, Riemann worked with both Wilhelm Weber and Johann Benedict Listing within the physics department; he eventually developed a skillful ability in theoretical physics [1, 3]. Riemann’s doctoral thesis investigated the geometric properties of analytic functions of complex variables; this applied the theories of topology to the study of analytic functions [3]. At the recommendation of Gauss, Riemann was appointed a position at the University of Gottingen; this post allowed him to work on a habilitation degree which would qualify him to become a lecturer at the university [1, 3]. To obtain this qualification, Riemann wrote a dissertation in which Riemann discusses the convergence of Fourier series [1, 3]. As a preliminary of this investigation, Riemann includes a definition for the integrability of a function [3]; nowadays, this condition is known as the Riemann Integrability.
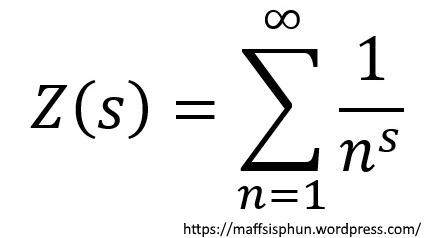To complete his qualification, Riemann presented a spectacular lecture on the topic of geometry [1, 3]. In this lecture, Riemann proposes a mathematical model for real space [3]; nowadays, this is referred to as a Riemannian Manifold [1]. In 1857, Riemann was awarded the position of Extraordinary Professor at the University of Gottingen providing him with some financial security [1, 4]. In July of 1859, Riemann was elected a member of the Berlin Academy of Sciences; at the request of the Academy, Riemann corroborated the research of Academy regarding the prime counting function [3]. This investigation prompted him to explore the implications of what is known today as the Riemann Zeta Function [3]; the Riemann Hypothesis states that the real part of all complex roots of this function are one-half excluding trivial roots.
Final Years.
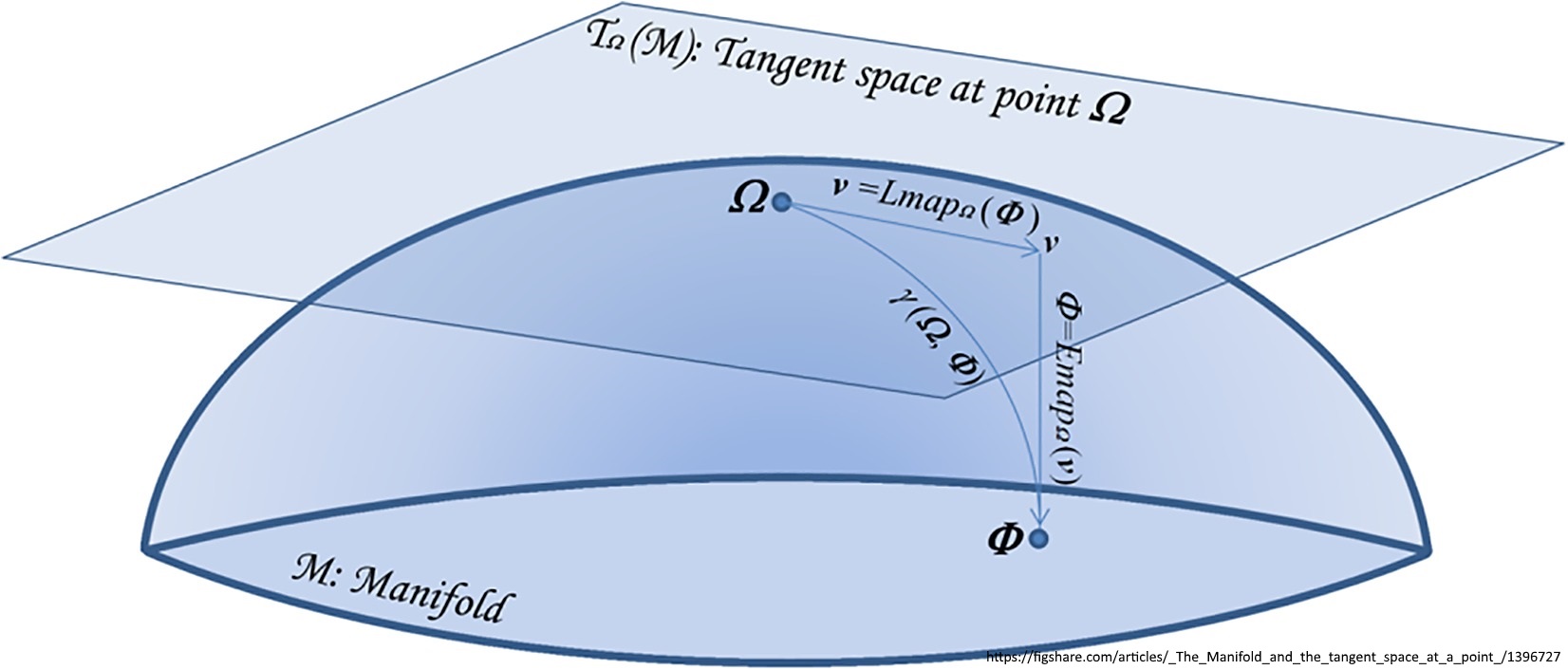In 1862, Riemann married Elise Koch and had a daughter [1, 3, 4]. Shortly after marrying, Riemann developed tuberculosis; consequently, Riemann moved to the warmer climate of Italy hoping to combat the progress of the disease [2, 3]. Riemann spent the remainder of his life periodically returning to Gottingen; until on July 20, 1866, Riemann died in Selasca, Italy at 39 years of age [1, 2, 4]. Riemann is known for his unique perspectives on geometry; he permitted space to have arbitrary dimension [2]. He contributed greatly to the theories of complex functions, complex analysis, and number theory [1]. His theories inspired many of his contemporaries to undertake much of his unfinished works [3]. Indeed, his work would become the foundation for Albert Einstein's theory of relativity [1, 2, 3, 4].